LearningArithmeticinthe21stCenturyMakeshiftCrew

LearningArithmeticinthe21stCenturyMakeshiftCrew

To-Do

Develop principled arguments (backed up by: research, empirical findings, your personal experience, hypotheses about resulting cognitive developments, and the topics discussed in class) which of the four positions YOU will favor!

Principled argument which of the four positions YOU will favor!

Assignment #2: Learning Arithmetic in the 21st Century with Option 4

          Our proposed plan for the Boulder Valley School district is for students to learn how to do arithmetic and calculations by hand while simultaneously learning how to use a calculator for those same tasks. The goal would be for children to develop enough proficiency that they could perform any calculation without the use of a calculator or other device if necessary. However, another goal is for students to understand how calculators can help them solve problems and be used in every day life. If technology is used intelligently in a classroom, it can be very beneficial to students' learning. While students need to have a firm grasp of mental math and the ability to use paper and pencil to solve problems, the use of calculators in combination with this strong background can make math more interesting, potentially spurring more interest in engineering fields.

Positive Aspects of Calculator Use
           Since calculators greatly reduce the time needed to perform calculations, using them allows students to explore more complicated and perhaps more applicable problems. In this way, the use of calculators could help students appreciate math more, because they see that it is actually useful in the real world. Indeed, studies have shown that students who use calculators have a better attitude towards math than those who do not use calculators (Hembree). Forbidding calculator use at all times will simply create frustration in the students who realize that calculators are everywhere. If students are given some problems for which they are allowed to use a calculator, they will hopefully realize that their teachers are aware of this. Also, using calculators can help improve mathematical understanding and problem-solving skills (Ellington). For certain problems, students can focus on how to set up the solution without needing to worry about the hand calculations that need to be done. Developing this skill is important for future math classes and even careers.

           Calculators may be the key to inspiring more interest in mathematics. Studies done in classrooms in Great Britain showed that students who used only calculators to learn arithmetic successfully invented their own methods of solving the same problems on pencil and paper (Waits). Since mathematics can be boring for some students, calculators can perhaps be used to create projects that spark more interest than pencil and paper methods do. These projects must be constructed carefully, and feedback from students should be frequent. As a child, word problems that adults come up with are often boring and inaccessible. Creating projects that are challenging but still accessible for students could give them confidence in their abilities to use math to solve relevant problems. Confidence is important, because once it is lost, interest will most likely follow.

Negative Aspects of Calculator Use
           Even though calculators can be used in almost every instance of computation, it is still important for students to know how to do all of these computations in their head and by hand. One reason is that basic arithmetic is the foundation for many other mathematical skills later in school. For example, long division may seem tedious once children learn that they can use a calculator to divide two numbers, but understanding the process is necessary when doing long division on polynomials, which cannot be done on simple calculators. If students had not already learned the concept of long-division, it would be much more challenging to grasp. Furthermore, doing arithmetic without the use of a calculator helps students develop intuition and estimation skills. It is useful to be able to glance at a grocery receipt and know if it is approximately accurate, to be able to leave a tip at a restaurant, adjust fractions in a recipe, and many other simple tasks. Students should be able to comfortably handle these situations on their own, since a calculator would hinder their ability to solve problems more than it would help them.

           It is important to avoid dependence on a calculator by ensuring that students practice mental math or pencil and paper math to the point where they cannot get it wrong. As students get more advanced in math, they will find that graphing calculators can be substituted for understanding in many instances. However, as students embark upon more advanced level college classes, they will quickly find that the calculator is an useless tool without the ability to understand how to solve a problem (Nelson). In advanced classes, it is expected that students can recognize patterns in proofs and data, which is very difficult without a strong base in mental math. Even though one can pull out a graphing calculator and plot a logarithmic curve on their own time, in a fast-moving lecture it is important to know the concept by heart and understand what it means. This and similar instances in a lecture can mean the difference between understanding and frustration. In chemical engineering courses, complex differential equations are reintroduced to help solve process control problems and describe the transfer of momentum, mass, and heat. In these classes, if a student is not completely comfortable with all proof techniques learned in earlier math classes, they will be lost, and there is usually not enough time to learn the math in combination with the chemical engineering concepts. While chemical engineers use programs such as Matlab to quickly simulate control situations in complex systems on computers, they would not be able to complete any assignments with Matlab without a firm grasp of the underlying mathematical concepts. Unfortunately, calculators do not have all the answers since the engineer is the one responsible for choosing what conditions to simulate based on the real world.

           In addition, mental math and calculator-free math builds confidence. It has been seen that for students who are able to quickly come up with an answer for problems mentally such as "Estimat[ing] a 40 percent discount on a $54.90 item" or finding "the hypotenuse of a right triangle with legs 10 and 24" are more readily able to grasp new concepts in math (Rubenstein). Kumon, the largest educational company in the world, achieves its success in teaching math and reading based on these principles. With 2.57 million students enrolled in 1998, Kumon has been teaching students a love for learning by practicing math at high speed until students are able to get every math problem correct (Adam Smith Institute). Kumon has had the same philosophy since 1958: no calculators (Davidson). "With our emphasis on individualized learning, your children will become independent, self-reliant, and motivated to learn on their own" (Kumon). By moving at the student's pace and never advancing until the current concept is mastered, Kumon helps slower students catch up without being intimidated, and allows advanced students move on to more difficult topics. Kumon students are the ones doing calculus in 5th grade. And they do it all without calculators. Though it is not recommended that students go through school without being exposed to calculators, Kumon's lesson is clear: students can achieve a high level of self confidence by learning to do the math on their own.

Division of Using a Calculator (when to use and when not)
           We propose a mathematics curriculum that involves instruction and practice both with and without calculators. Since students' have a short attention span, this could also help break up the monotony of some lessons. Students should be given some exercises that require them to do arithmetic mentally and on paper in order to master the concepts analytically. Certain problems, especially word problems or calculations designed to explore larger numbers or patterns, should be done with the aid of a calculator. In using a calculator, students should be able to reinforce their answers by reasoning as a verification to detect inaccuracies and mistakes like skipping steps or entering numbers wrong in the calculator (Miller). An example is in graphing the function y = x^(2/3) on a TI-82 calculator. Figure 1a shows the error produced on a calculator by directly placing the equation in the plotter. If the student understood how the function worked, they would have noticed that the calculator failed to completely graph the function. They would then have corrected this error caused by the limitations in the TI-82 calculator by re-entering the function as either y = (x^2)^(1/3) or y = (x^(1/3))^2. The graph of the function would then be correctly displayed as shown in figure 1b (Zheng 6).

 

Works Cited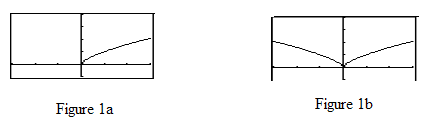
Adam Smith Institute. "56: Sum Success: Origins of the world's biggest education company." Around the World in 80 Ideas Blog. 2002. http://www.adamsmith.org/80ideas/idea/56.htm. 6 September 2010.

Davidson, Alex. "Remedial Math." Forbes. 2 March 2009. pg 95. http://kumonoftucsoncasasadobes.com/Documents/KumonInForbesMar09.pdf. 6 September 2010.

Ellington, A.J. (2003). "A Meta-Analysis of the Effects of Calculators on Students' Achievement and Attitude Levels in Precollege Mathematics Classes." Journal for Research in Mathematics Education: 34, 433-463.

Hembree, R & Dessart, D.J. (1986). "Effects of hand-held calculators in precollege mathematics education: a meta-analysis." Journal for Research in Mathematics Education: 17, 83-99.

"Kumon: Preschool through 12th Grade." Kumon. 2010. http://www.kumon.com/WhyKumon.aspx. 6 September 2010.

Miller, Maria. "Using Calculator in Elementary Math Teaching." Homeschool Math. 2010. http://www.homeschoolmath.net/teaching/calculator-use-math-teaching.php. 6 September 2010.

Nelson, Ryan. "The Calculator as a Crutch: Letter to the Editor." 9 September 1999. New York Times: 4.

Rheta N. Rubenstein, Rheta N. "Mental Mathematics beyond the Middle School: Why? What? How?" Mathematics Teacher 94 No 6 442-6 S 2001.

Waits, Bert K., Demana, Franklin. "Calculators in Mathematics Teaching and Learning: Past, Present, and Future." 2000. Yearbook (National Council of Teachers of Mathematics) 2000: 51-66.

Zheng, Tingyao. "Impacts of Using Calculators in Learning Mathematics." State University of New York College at Fredonia. http://www.atcminc.com/mPublications/EP/EPATCM98/ATCMP015/paper.pdf. 6 September 2010.

---

Authors of this document are the Makeshift Crew:

Albierto Aranda
Andy Truman
Anne Gatchell
Ho Yun "Bobby" Chan
Kyla Maletsky